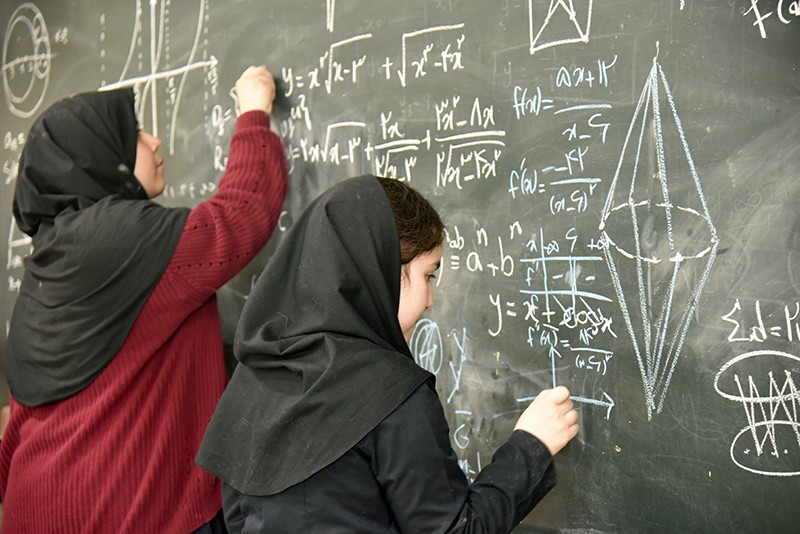Iranian girls were inspired to take up mathematics as a result of Maryam Mirzakhani’s success
12 June 2020 – Maryam Mirzakhani broke into the exclusive club of top mathematics prizewinners in 2014. It was a momentous occasion: whether because of committee biases or the gauntlet of systemic obstacles and social pressures, no woman had previously won the discipline’s most coveted award, the Fields Medal, established in 1936. This short video was produced by Wired magazine shortly after she won the prize:
Now, it seemed that the pipeline of female talent was finally beginning to deliver. George Csicsery’s Secrets of the Surface is the first feature-length film on Mirzakhani. It celebrates how she broke multiple glass ceilings – she was also the first Iranian to win the prize. And it strikes a satisfying balance between her magnificent mathematics and her human story, including her untimely death from cancer in 2017, at the age of 40.
The film traces Mirzakhani’s roots and legacy, interviewing current students and teachers at the school for intellectually gifted girls she attended in Tehran. She did not show a particular interest in maths early on. Instead, she devoured books, dreaming of becoming a writer. But her aptitude for numbers showed: classmates joked that when presented with a problem in a maths lesson, she took pleasure in solving it four different ways.
As a writer and video producer, that was what fascinated me. As an 8-year-old, Maryam Mirzakhani used to tell herself stories about the exploits of a remarkable girl. Every night at bedtime, her heroine would become mayor, travel the world or fulfill some other grand destiny. Her chief goal was simply to read every book she could find. She also watched television biographies of famous women such as Marie Curie and Helen Keller, and later read “Lust for Life,” a novel about Vincent van Gogh. These stories instilled in her an undefined ambition to do something great with her life — become a writer, perhaps.
As a mathematics professor at Stanford University she still wrote elaborate stories in her mind. The high ambitions did not change, but the protagonists did: they were hyperbolic surfaces, moduli spaces and dynamical systems. In a way, she said, mathematics research feels like writing a novel. “There are different characters, and you are getting to know them better,” she said. “Things evolve, and then you look back at a character, and it’s completely different from your first impression.”
She followed her characters wherever they took her, along story lines that often take years to unfold. Petite but indomitable, Mirzakhani has a reputation among mathematicians for tackling the most difficult questions in her field with dogged persistence. “She has a fearless ambition when it comes to mathematics,” said Curtis McMullen of Harvard University, who was Mirzakhani’s doctoral adviser.
Inspirational performance
In 1994, Mirzakhani and her best friend, Roya Beheshti – now a mathematician at Washington University in St. Louis, Missouri – became the first women in the Iranian delegation to the International Mathematical Olympiad. Mirzakhani returned the next year, and gained a perfect score. Her exceptional performance made her a national celebrity, and inspired other Iranian girls to study mathematics.
Graduating from Sharif University of Technology in Tehran, she went on to study for a PhD at Harvard University in Cambridge, Massachusetts. (A move that would these days be extremely difficult, given US President Donald Trump’s restrictions on travel to the United States from Iran and other countries.) In her thesis, Mirzakhani solved a central problem about curves on Riemann surfaces, a subject with a 150-year pedigree. To describe this and other achievements, the documentary switches from biographical to educational mode, with elegant animations.
Billiard-ball breakthrough
Soon after her earning her PhD, Mirzakhani became a faculty member at Stanford University in California, where she made several other breakthroughs with various collaborators. In one major result, she and Alex Eskin of the University of Chicago in Illinois studied the chaotic dynamics of billiard balls.
In the film, many collaborators and peers describe a problem solver with the indomitable spirit of a marathon runner. Some fight back tears as they recall working alongside her. Their accounts are greatly enriched by interviews and other footage of Mirzakhani shot for a series of videos on the four winners of the 2014 Fields Medals.
Csicsery has carved a niche as a maker of compelling films about mathematicians, starting with the brilliant 1993 N is a Number, on Paul Erdős, the most productive mathematician of the modern era and a perennial couchsurfer. Csicsery’s latest work celebrates the importance of female role models by showing the impact of Mirzakhani’s short but extraordinary life on today’s young women. But it never directly addresses the big question: why has no other woman ever won a Fields Medal (and only one, Karen Uhlenbeck in 2019, has received the Abel Prize), and what can be done to change this?
Secrets of the Surface – Director: George Csicsery Zala Films (2020) http://www.zalafilms.com/secrets
* * * * * * * * * *
Her math
Her math is not easy. I downloaded a host of material from the Fields Medal site and despite a science and (a bit) of a math background I got lost. Erica Klarreich, an American mathematician, journalist and science popularizer who is in one of my social media groups, provided this:
Mirzakhani became fascinated with hyperbolic surfaces — doughnut-shaped surfaces with two or more holes that have a non-standard geometry which, roughly speaking, gives each point on the surface a saddle shape. Hyperbolic doughnuts can’t be constructed in ordinary space; they exist in an abstract sense, in which distances and angles are measured according to a particular set of equations. An imaginary creature living on a surface governed by such equations would experience each point as a saddle point.
It turns out that each many-holed doughnut can be given a hyperbolic structure in infinitely many ways — with fat doughnut rings, narrow ones, or any combination of the two. In the century and a half since such hyperbolic surfaces were discovered, they have become some of the central objects in geometry, with connections to many branches of mathematics and even physics.
But when Mirzakhani started graduate school, some of the simplest questions about such surfaces were unanswered. One concerned straight lines, or “geodesics,” on a hyperbolic surface. Even a curved surface can have a notion of a “straight” line segment: it’s simply the shortest path between two points. On a hyperbolic surface, some geodesics are infinitely long, like straight lines in the plane, but others close up into a loop, like the great circles on a sphere.
The number of closed geodesics of a given length on a hyperbolic surface grows exponentially as the length of the geodesics grows. Most of these geodesics cut across themselves many times before closing up smoothly, but a tiny proportion of them, called “simple” geodesics, never intersect themselves. Simple geodesics are the key object to unlocking the structure and geometry of the whole surface.
She had these huge pieces of paper on the floor and spent hours and hours drawing what look to me like the same picture over and over. Yet mathematicians couldn’t pin down just how many simple closed geodesics of a given length a hyperbolic surface can have. Among closed geodesic loops, the simple ones are miracles that effectively happen zero percent of the time. For that reason, counting them accurately is incredibly difficult: if you have a little bit of an error, you’ve missed it.
In her doctoral thesis, completed in 2004, Mirzakhani answered this question, developing a formula for how the number of simple geodesics of length L grows as L gets larger. Along the way, she built connections to two other major research questions, solving both. One concerned a formula for the volume of the so-called “moduli” space — the set of all possible hyperbolic structures on a given surface. The other was a surprising new proof of an old conjecture proposed by the physicist Edward Witten of the Institute for Advanced Study in Princeton, N.J., about certain topological measurements of moduli spaces related to string theory. Witten’s conjecture is so difficult that the first mathematician to prove it – Maxim Kontsevichof – was awarded a Fields Medal in 1998 in part for that work.
Solving each of these problems would have been an event, and connecting them would have been an event. Mirzakhani did both.
Mirzakhani’s thesis resulted in three papers published in the three top journals of mathematics: Annals of Mathematics, Inventiones Mathematicae and Journal of the American Mathematical Society. The majority of mathematicians will never produce something as good – and that’s what she did in her thesis.